June 2015 Archives

On Monday, June 29, 2015, California State Assembly Bill (AB) 525 was considered by the Senate Business, Professions and Economic Development Committee.

If passed by the Senate, AB 525 will amend the existing California Franchise Relations Act (Business and Professions Code §§ 20000 - 20010) ("CFRA") by expanding protections for existing franchisees. See my previous blog post for a discussion of the amendments to the CFRA that would be created by AB 525.

While the Senate has made some minor clean-up amendments to AB 525, none of them substantially change the character of the bill or the amendments that it would create to AB 525.

The International Franchise Association ("IFA") has mounted a significant challenge to AB 525, having sent to its California-based members an email urging them to contact their elected officials and voice opposition to the bill.

From the IFA's email:

This bill in its current form places the basic tenets of the franchise contract at risk, which ultimately would harm franchisees, franchisors and consumers in California. This is a dangerous precedent, as this legislation could damage the reputation of local franchise business owners by reducing their brand standards. The unintended consequences of this legislation will also drastically increase incentives for litigation.

As an interesting example of politics making strange bedfellows, one of the key sponsors to AB 525 is the Service Employees International Union (SEIU).

The SEIU is the driving force behind the National Labor Relations Board's (NLRB) push to hold franchisors liable for their franchisees' employees as "joint employers," which push is widely considered to be a significant threat to the franchise industry as a whole.

According to Catherine Monson, CEO of FASTSIGNS, AB 525 is part of the SEIU's "coordinated attack on the franchise business model."

To assist the IFA in its campaign to defeat AB 525, you can follow this link: https://www.votervoice.net/FRANCHISE/Campaigns/39986/Respond

This is the first of a three articles intended to raise investment care sensitivities. They are offered as a gesture of concern about what I see happening to people who invest in new and in old/over the hill franchise opportunities.

The purpose of the deceits practiced by these two groups of franchisor companies is, in the first instance, to portray itself as the investment quality equivalent of an already proven franchise concept/model, and, in the second instance, to portray itself as still being as good an investment vehicle as it once may have been but no longer is.

Is it possible for franchisors to say to prospective franchisees that profitability information is not provided when they do they provide profitability information?

It is also a very logical question to ask whether a franchisor can provide false or misleading profitability information so long as a warning is also given that there is no guaranty that you will achieve profitability and that you assume the risk of profit or loss and that there are risks.

Since risk is inherent in any investment, warnings about risks do not include or excuse risks that are produced by false statements made to induce the investor to part with his money in the first place.

How does someone who is thinking of investing in a franchise go about vetting the numbers?

This is the hottest button issue in disclosure, because assurances of potential profit are the most effective way to sell a franchise. Only an imbecile buys a franchise because of the bullshit. Real people want to know whether they will make a profit and how much, and how long it will take to reach profitability, and how much it will cost to get there.

Many years ago I published a suggestion in the ABA Franchise Newsletter that, since every franchise agreement empowers the franchisor to receive copies of periodic financial information and tax returns generated by its franchisees, compiling that information ought to be made mandatory, and that information ought to have to be disclosed in a way that displays how the franchisees are really doing.

This would include grouping franchisee performance information by levels of profitability (top third, middle third, bottom third), by region of the country, and by showing seasonality if seasonality is a significant factor. I also suggested that the information could be handled in such a way as to eliminate the impact of different franchisees treating various expenses differently for accounting or tax purposes.

If that were to happen, people might be able to appreciate at a higher level of quality just what the performance expectancies might be.

Franchisors, of course, howled at the thought. Why, if that were done, people might be able to see whether your franchise system was more profitable for its franchisees than someone else's franchise system, and the lower performing franchise systems would have a harder time selling franchises. DUH! That's one of the best reasons for doing it!

Since a potential franchisee will not receive that quality of prospective financial performance information, it remains necessary to have access to a fairly high level of sophistication in dealing with what is provided.

This article is intended to help to deal with the garbage that is thrown at you now.

Reading this article does not make you my client, and there are many other things that must be accounted for than just the questions raised here. But you can take this to your lawyer or accountant/financial planner, and maybe it will help you both better understand what you are being handed.

Are there Franchise Consultants? No.

Let's get started. Start by first asking yourself who it is that you are dealing with -- who is behind the face you are looking at when you are dealing with the person who is providing you with initial information about a franchise opportunity? If that person identifies himself/herself as a 'FRANCHISE CONSULTANT', you know you are dealing with a liar.

There is no such thing as a franchise consultant who sells franchises. While many people do that, they are not consultants in any functional meaning.

The reason for that is that they only get paid for selling franchises -- usually by a commission. That's a salesperson, not a consultant. And even if they were consultants, they aren't working for you to help you.

If you aren't the only one paying them for assistance, they are salespeople. When they tell you that they are there to help you get the right deal for your circumstances, that is total bullshit. They are there to sell you a franchise -- any franchise that will pay them a commission. You have to be extremely cynical about everything you are told.

Doubt everything until it is proven -- and you need to know what proof looks like.

What they call proof usually isn't. In once case in my office, the franchisor sales person claimed to be a vice president when he wasn't even an employee of the company. He was an outside salesman. You have to doubt EVERYTHING.

Item 19 Traps

a) - No Item 19 earnings claim is a Lie

Liar Number One tells you in Item 19 of the UFOC that the company does not give out earnings claims and that no one is authorized to provide earnings information in connection with the sale of its franchises.

This is an obvious lie for several reasons. The first reason is that the only reason to make an investment is to make a profitable return on that investment. If there is no representation of profitability potential/earnings potential, then there cannot be any reason on earth to make an investment in their franchise.

The second reason is that every one of these franchise companies does make earnings claim information to every potential franchise purchaser. The earnings claims information isn't in Item 19 of their UFOC, but it is provided by other means.

Among these other means you will see one or several of the following:

The franchise sales person provides the information either orally, on a blackboard, on a random piece of scrap paper/cocktail napkin/table cloth (and if you hang on to that piece of paper you may have a great exhibit when things later don't go as you thought they should).

b) Franchisee Verifications cannot be relied upon

The franchise sales person invites other franchisees to come appear at a meeting of franchisee prospects to tell of their experience as a franchisee. These folks always tell about their own wonderful financial performance. The presentation is intended to tell you that this is what you can expect if you buy the franchise.

The selected franchisee spokespeople are either all very successful or just shills lying through their teeth. In some instances these shills also receive a 'commission' on every franchise fee taken from each person attending that meeting who buys a franchise.

There are probably some successful franchisees in the system, but the point is that you are being provided with information that the franchisor denies providing to you, and that the information -- since it is denied it was ever given anyway -- is never reliable.

If you do buy the franchise, you will see in the franchise agreement an Acknowledgement clause that says that you agree that you were never given any earnings claims information.

If you sign that agreement knowing that you were in fact given earnings claim information, you may be out of luck even getting it admitted into evidence in court or in arbitration of any fraud claim you may later seek to assert.

Some courts are allowing the Acknowledgement clause to exonerate misrepresentation, making the defrauded franchisee seek reversal of those rulings in courts of appeal. That adds time and expense to obtaining relief.

You are provided, as is required, a list of the names, addresses and telephone numbers of all the franchisees of that franchisor so that you can contact them as part of your investigation/due diligence before you buy the franchise, to see what kind of experience they are having with the franchise.

But the franchise sales person steers you to certain franchisees. These will always say they are making tons of money and are just thrilled with the franchisor who provides great training and support. If you do buy the franchise, the sales person usually gives them a percentage of the sales commission, or they receive some other form of consideration for their assistance.

The franchisor's position is that, notwithstanding the steering, they have no part in providing you with earnings claims information. If you buy that you are too stupid for words. While it may in some strict sense be true that someone else, not the franchisor, provided you with the information, the fact is that the information was provided to you through the efforts of someone affiliated with the franchisor.

c) Financial Assistance for SBA Loans, but no Item 19

The franchisor who claims not to provide earnings claims information to persuade you to buy the franchise, does offer to assist you in seeking an SBA loan. The loan requires that you prepare a 'business plan' (another work of sheer fiction). The business plan requires a pro forma operating statement and balance sheet statement to show the lender what you expect by way of financial performance of the business for which you are borrowing the money.

The franchisor either provides you with information to use in preparing the business plan, including the financial pro forma, or steers you to a 'consultant' to assist you and the consultant provides financial pro forma information that the consultant obtained from the franchisor.

Then of course, you take your business plan to the franchisor for review, before submitting it to the SBA lender, and the franchisor tells you that you 'did a good job' or that 'your numbers are reasonable', vouching for the numbers/earnings claims information.

The lying franchisor's position is that they provided nothing and that you got that only from some third party, not from the franchisor. The franchisor's back up position is that they didn't provide you with the information in order to get you to buy the franchise. They just provided information to help you get a loan.

DUH! And -- I actually heard a franchisor person testify to this once -- the ultimate fall back position if the information is false is that the false information wasn't provided to you, but was provided to the lender.

There are other ploys by which franchisors who state in Item 19 of their UFOC that they don't give out earnings claims financial information actually do in some fashion provide it.

The fact is that it is almost never true that they don't provide earnings claim information, and if they provide it while insisting that they are not providing it, they are lying to you and you need to run like hell, not buy the franchise.

As always, you can call me, RIchard Solomon, at 281-584-0519.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

Through effective trade associations and lobbying efforts, during the last century automobile dealer franchises in the United States convinced state governments to give them significant protection against commercial abuse or unfair dealing by the manufacturer or supplier franchisors.

Franchisees in other industries could learn from that example.

The strength of the laws protecting dealer franchises was demonstrated by a recent New York court decision in Audi of Smithtown, Inc. v. Volkswagen Group of America, Inc. .

The case was brought by one set of Audi dealers charging that Audi's wholly-owned subsidiary, VW Credit, Inc. discriminated in favor of new dealers to the detriment of the incumbents who brought the case. At issue were incentives that VW Credit put in place for dealerships to purchase vehicles returned by customers at the end of their leases.

(For example, if Joe Brown leases an Audi Quattro for 3 years, the vehicle is owned by VW Credit during the lease, so at the end of 3 years, VW Credit has a "pre-owned vehicle" to sell. )

The incentive programs were based on the proportion of returning off-lease vehicles that a dealership purchased. However, since incumbent dealerships had more leases, they had more opportunity than new dealers to benefit from the incentives.

To level the playing field, VW Credit automatically granted new dealers a more favorable level of available discounts and bonuses (known as "Champion Level") for the first three years of the dealership. While this program seems to have a logical business justification - making it easier to open a new dealership, which increases Audi's presence in the local market -- the Court ruled instead that it constituted price discrimination against the incumbent dealers.

New York's law provides: "It shall be unlawful for any franchisor . . . [t]o . . . sell directly to a franchised motor vehicle dealer . . . motor vehicles . . . at a price that is lower than the price which the franchisor charges to all other franchised motor vehicle dealers." N.Y. Vehicle & Traffic Law § 463(2)(aa).

Audi argued that VW Credit is not a "franchisor" under the statute and therefore no violation could have occurred. The dealers had that covered, however, because in 2008 the New York legislature amended the statute to add references to "captive finance sources" so as to prohibit a motor vehicle franchisor from using "any subsidiary corporation, affiliated corporation, captive finance source, or any other controlled corporation, company partnership, association or person to accomplish what would otherwise be unlawful conduct under this article on the part of the franchisor." N.Y. Vehicle & Traffic Law § 463(2)(u).

The New York laws prohibiting price discrimination and the use of shell entities to get around the law are similar to those in others states that protect car dealers in their relationships with their franchisors.

In Maryland, for instance, the law requires manufacturers to act honestly and observe reasonable commercial standards of fair dealing in performance or enforcement of the franchise agreement. They are also not allowed to:

1. Coerce dealers to do something not required by their franchise agreements, or make them agree to material modifications (for instance, changes to their purchasing or performance requirements), unless the changes apply to all other Maryland franchisees of the same manufacturer.

2. Stop a dealer from offering other manufacturers' products at the same facility through a franchise agreement granted by another manufacturer.

3. Require a material change to "the dealer's facilities or method of conducting business if the change would impose substantial financial hardship on the business of the dealer."

4. Require a franchisee to adhere to performance standards unless, as applied, they are "fair, reasonable, equitable and based on accurate information."

5. Refuse to permit an individual to be the responsible person of the dealer "unless the individual is unfit due to lack of good moral character or fails to meet reasonable general business experience requirements" -- and the manufacturer has burden of proving unfitness.

6. Unreasonably withhold consent to a request to transfer a franchise, and an aggrieved franchisee has a right to an administrative remedy to contest a manufacturer's refusal to consent.

7. Terminate the dealership for any reason without payment to the dealer of compensation for various types of assets and franchise-specific improvements.

8. Require the dealer to reimburse it for attorneys' fees in any dispute involving the franchise.

Maryland Transp. Code Sections 15-206.1 through 15-212.2. In addition, aggrieved franchisees have a right to bring an action for damages and reasonable attorneys' fees incurred in vindicating their rights. Id. at Section 15.-213.

These statutes are not a cure-all for auto dealers who fail to properly execute their responsibilities. And, in any event, the 2009 bankruptcy proceedings of General Motors and Chrysler show that extreme economic circumstances can trump state statutory rights.

But overall, the various state laws protecting automobile dealers show the advantages of a century-old industry, widely dispersed, and generally liked in their local communities.

The auto dealers have been able to put their case to their state representatives and win some good protections. This is an example franchisees in other industries could learn from.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

Vicarious Liability in General

Nearly every franchisor has suffered threats of litigation based upon the actions of its franchisees. These threats are not necessarily limited to claims stemming from the franchisees' delivery of services (from mold remediation to serving tasty food which does not result in gastro-intestinal distress), but may extend to those arising from the franchisees' employment decisions.

Generally, the franchisor defends on the basis that it does not direct the day-to-day actions of its franchisees, and the franchise relationship by definition is an independent contractor relationship, rather than an agent-servant relationship.

A recent case out of Washington recognizes this principle, but with a current technological and statutory gloss.

Robo Calling by Franchisee using Franchisor Mandated POS

In this case, a sizable Domino's Pizza franchisee (Four Our Families, Inc.) hired a telemarketing firm (Call-Em-All LLC) to help increase sales, and that firm engaged in "robo-calling" to offer pizza delivery specials.

A class action lawsuit was filed claiming violations of the federal Telephone Consumer Protection Act (47 U.S.C. Section 227 et seq.) and a Washington statute (Revised Code of Washington Section 80.36.400, "WADAD," governing "automatic dialing and announcing devices"). The putative class action plaintiffs claimed that they had received unsolicited auto-calls offering pizza deals from Domino's, and those calls were made without their prior consent.

The plaintiffs claimed that the franchisor was liable for the franchisee's actions under the general contract provision granting the franchisor the right to control advertising and marketing decisions. The franchisor denied that it was involved in the robo-call efforts.

In support of their claim against the franchisor, the plaintiffs pointed out that the telemarketing firm had advertised its services at a Domino's national convention, and also had used the PULSE telephone system (which Domino's Pizza requires the franchisees to use, and which is capable of producing lists for "ADAD" calling).

Ruling By Court - No Franchisor Liability for Mandating POS

The federal district court granted the franchisor's summary judgment motion and denied class certification. The court recognized that the franchisor did not conduct a robo-calling campaign itself, participate in the franchisee's campaign, or direct that franchisees conduct such a campaign.

The court stated that the "mere fact that Domino's requires franchisees to participate in marketing campaigns does not somehow mean that any franchisee's illegal use of an [automatic dialing and announcing device] is imputed to the franchisor."

This is just one more example of an effort by the plaintiffs' bar to hold franchisors accountable for the actions of franchisees.

Franchisors should take heed, and zealously defend the independent contractor relationship and the realities of the relationship.

In addition, franchisors would be well advised to inquire into the availability of insurance for these and similar claims.

The court's order may be reviewed by clicking here.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

The franchise industry is on the verge of losing a battle regarding employer liability, as old arguments fail to address new opponents.

Whatever your position on the Obama view of franchising, the reality is that Executive Branch officers are powerful and have wide discretion. As such, franchisors ignore their views at great peril.

A recent article by attorney Harold Kestenbaum made the case that state legislators are able to determine how "employer" is defined under federal law. Presumably Mr. Kestenbaum was being facetious in demonstrating the failure of the International Franchise Association and others to address the concerns of the NLRB and US Dept of Labor, but the large number of industry players who took the article as a serious comment are indicative of just how clueless the franchise industry remains today.

David Kaufmann may lack Mr. Kestenbaum's sense of satire, but Kaufmann's article in the Spring 2015 Franchise Law Journal is a serious attempt to lay out the basis for the threat and a socio-legal defense of the existing jurisprudence. The article is worthy of more serious consideration than can be dealt with in a brief BMM post, and is the starting point not only for an attack on the Obama administration viewpoint but also for a frank discussion of why David Weil is having success.

Kaufmann is blunt in identifying the root cause of the problem, and it is to this point that my comment is addressed.

American political discourse has gone thru many cycles in our history, and we are now in an environment similar to the great Progressive Era of a century ago. As Kaufmann realizes, the impetus is also similar to the last cycle: just as the Gilded Age led to a reaction against inequality of wealth and political influence, the wake of the 2007 financial collapse has led to a reevaluation of economic changes in recent decades.

Most relevant to our concerns, the gap in wages for those at the bottom of the ladder stands out in sharp contrast to the wealth of those at the top. A century ago, this led to some action at the federal level (such as the Sherman Act), but most of the activity was at the state level, and union organizing was in its infancy.

Much as the encyclical Rerum Novarum (1891) led to calls for a "living wage" in the 1920s, religious leaders today are calling attention to the moral aspects of how QSR workers are being treated in 2015.

Politicians are by nature attuned to popular sentiment, whatever the century. Theodore Roosevelt sought to follow Herbert Croly's prescription to achieve "Jeffersonian ends through Hamiltonian means." There are significant differences between Croly and Weil, and Obama is no TR. Yet even starting from a conservative viewpoint (as did Croly and TR), the remedy of David Weil is not without foundation in American politics or jurisprudence.

While the Great Depression marks a break from the Progressive Era, the legislation which resulted (including the creation of the NLRB) was very much informed by the progressive values of the first three decades of the century.

New Deal legislation coupled with the effects of World War II led to the locus of power shifting to Washington and in the wake of Chevron and the practical realities of statutory implementation, our time is one in which the state governments are bit players (with some exceptions such as Eric Schneiderman).

Kaufmann says that if the Obama viewpoint takes hold, then franchisors would be on the hook for increased costs due to the need to comply with Wage & Hour mandates. Costs would increase and franchising could not survive, says Kaufmann.

No doubt Weil would agree and explain that is precisely the point. Costs would increase as employees would have to be paid in accord with statute, and the ability to go after a "deep pocket" would mean that businesses would have to either comply with the law or be sued.

In fact, this is precisely the logic behind NY Attorney General Schneiderman's pursuit of pizza franchisors Domino's and Papa John's.

Kaufman realizes the problem, but he does not propose a solution and spends most of the article formulating a legal argument regarding why "joint employer" theory should not apply to the franchise industry.

Kaufmann makes a respectable case on this latter point, but that is not going to solve the underlying problem, since this is an issue which is every bit as much a public policy issue as a legal one-- and I would argue, more so.

Kaufmann is well aware of the underlying problem and his failure to address it is nothing to hold against him. But as he suggests in the beginning of his article, there is a populist and even a moral aspect to the Weil thesis which needs to be addressed.

We can debate whether the minimum wage was originally intended to be sufficient to live on (it was not), and whether the minimum wage was intended to be the support of full-time workers who are the head of household (again, it was not). We can also debate whether the top of the food chain is getting more than a fair share.

But at the end of the day, the franchise industry is left with a simple reality: the electorate perceives a gross inequality which needs to be rectified. And with every franchise owner who fails to abide by Wage & Hour statutes, and with every franchisor who turns a blind eye to wage theft and taxpayer subsidy of wages, the position of David Weil and the NLRB is going to get a more sympathetic ear.

It is time for the franchise industry to clean up its act, or at least to get through the next Presidential election cycle without suffering a disaster.

Morality aside, there are sound reasons why franchisors should take David Weil and the NLRB seriously-- answering not simply their legal arguments, but their economic arguments and the moral basis thereof.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

While most negotiators are aware of how quickly combat can threaten the success of a deal, few are aware of how concessions, the opposite of combat, can just as quickly ruin a negotiation.

In order to be a truly skillful negotiator, it's important to recognize the value of compromise. Choose your battles wisely- you will be amazed by how smoothly a negotiation progresses when both parties are willing push combativeness aside for compromise.

A key component of compromise is concession. Before you begin any negotiation, it's important to identify which components of the deal are non-negotiable, and which components you are willing to compromise on. While this step admittedly creates additional work for you, it will prove to be invaluable in the grand scheme of the negotiation.

What happens if you choose to skip this step and plunge headfirst into a deal? You're putting yourself (and your delegates) at risk. Lack of preparedness in a negotiation leads many negotiators to commit one of the cardinal negotiation sins: the unilateral concession.

Imagine that you are the main negotiator of a deal that has the potential to make your company a tremendous amount of money.

In your eagerness to begin the negotiation, you fail to identify which parts of the negotiation are open to compromise and which parts are ironclad. In a meeting with a representative from the opposing delegation, the following dialogue occurs:

Buyer: I'm sorry, but we've ultimately decided that we can't use you as our supplier. You're just too expensive.

You: There isn't a lot I can do about the price. Are you looking for a discount?

Buyer: Well...that's a good place to start. What can you give me?

You: Um...I can let you have... maybe 3%?

Buyer: Three percent? I'm afraid that's not enough.

You: I'm not authorized to go beyond 5%.

The entire deal has been threatened.

Blinded by panic, you've committed a major blunder: you've made a unilateral concession. The unilateral concession offers a solution that demands nothing in return.

The buyer recognized the fact that you had not considered the possibility of a discount, and he took full advantage of your unpreparedness. In an effort to save the deal, you cracked under pressure and offered a solution that will yield nothing for your delegation. While you've temporarily stopped the negotiation from crumbling, you've also made some costly mistakes:

1. You made no effort to find out what the buyer meant by saying that the price was too expensive. Too expensive compared to what? How much should it be? Has the buyer produced a cost calculation?

2. You negotiated the price instead of discussing total costs. In your panic, you made up your mind about the price without knowing what the buyer thought about the delivery time, volume, quality, warranties, performances, or other conditions.

3. By emphasizing the fact that you weren't authorized to go beyond 5% , you signaled that there was a greater discount that your boss could potentially approve.

Don't dwell on your mistakes- even the most skillful negotiators slip up. The most effective way to recover from your mistakes is to learn something from them. In next week's installment, we'll explore how you can recover from unilateral concession-making and get the negotiation back on track.

In the meantime, please feel free to share your own stories about your experience with concessions in the comments section.

Have you ever had a similar conversation? How did you recover?

This post is excerpted from Keld Jensen's book Power Bargaining: Adding Value to Commercial Negotiations.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

Business people and corporate counsel often seem not to pay much attention to their choice of dispute resolution mechanism when negotiating a contract. They often seem treat dispute resolution as just part of the contractual "boilerplate". If they give much thought to it at all, they probably plug in a choice of litigation jurisdiction or arbitration provision from a previous contract.

More likely, they don't consider the issue at all.

So the contract ends up saying nothing about dispute resolution. That leads to any disputes which arise under it being resolved by litigation.

As we'll see, that's likely the worst possible alternative.

This article reviews the basic characteristics of the most common dispute resolution mechanisms, so that business people and corporate counsel can make better informed choices on this issue.

It is helpful to consider negotiation, mediation, arbitration and litigation as lying along a continuum. The "negotiation" end of the continuum is characterized by values like acceptable results, flexibility and efficiency; the "litigation" end by values like risk of unacceptable results, expense and delay, and publicity.

Looked at that way, it's not hard to see which end of the continuum most businesses would prefer be on.

Negotiation

Let's start with negotiation. There is no mystery about what negotiation is: the parties compromise toward an agreed resolution of their dispute.

Negotiation has several advantages over other dispute resolution mechanisms. By definition, it produces an acceptable result. If both parties don't agree, there's no resolution.

Negotiation is infinitely flexible - it can accommodate anything the parties can dream up and agree on. In particular, it can accommodate solutions based on the parties' ongoing business interests, rather than just their strict legal rights and obligations.

While negotiation can certainly be complex, and therefore expensive and time consuming, it is usually more cost and time efficient than the alternatives. Negotiations, and their results, are generally confidential. That can be important in sensitive business contexts.

For all those reasons, negotiation is almost always the best dispute resolution mechanism.

There are good reasons most disputes are resolved by negotiated settlements. Businesses should always try to negotiate the resolution of a dispute (whatever their contracts say). They should never close the door on negotiation.

Not that negotiation is the perfect mechanism.

Again, by definition, it requires compromise.

So it's highly unlikely either party will get everything they want. It also involves significant uncertainty. Because both parties must agree on a solution, both have a veto over that solution. There's always the possibility the other side will "just say no" to any
reasonable resolution.

(To answer one client's question: No, the court won't order a party to agree to a resolution.)

In that case, negotiation just won't work. What then?

Mediation

Mediation is "just" negotiation facilitated by an agreed neutral, normally a trained and experienced mediator.

Ideally, if the parties are rational and competently advised, they should be able to negotiate a resolution of their own. But, sometimes that's just not the case.

Other times, something about the situation produces a negotiating impasse. In those situations, mediation can be a very
useful tool to achieve a negotiated resolution.

Mediation has essentially the same advantages (acceptability, flexibility, efficiency, confidentiality) and disadvantages (necessity to compromise, uncertainty) as negotiation. The differences are of degree rather than kind.

Most importantly, there is undoubtedly some kind of "magic" about mediation. It's hard to explain, and the reasons for it may be different in every case, but there is no doubt that the vast majority of commercial disputes which are mediated are
resolved through that process.

There is just something about involving a neutral in the negotiation that greatly facilitates resolution.

So, mediation is more certain to produce an acceptable result than negotiation.

One problem with mediation is that, not only must the parties agree on a resolution, they must also agree to mediate in the first place, and then on a mediator. Sometimes they can't, or just won't.

A practical downside of mediation, compared to negotiation, is that competent, experienced mediators are not cheap, nor readily available.

So mediation can be less cost and time efficient than negotiation. But, if it achieves an acceptable result, that cost and time may be well worth it.

Arbitration

With arbitration we move to a fundamentally different kind of dispute resolution mechanism. (This is why the mediation-arbitration hybrids can be so tricky.)

In arbitration the parties agree to give a neutral the power, not to facilitate an agreed resolution of their dispute, as in mediation,
but to impose a legally binding resolution on them, whether they agree with it or not.

Arbitration is essentially "private litigation." But, in the hands of experienced counsel, it can have important advantages over litigation. Arbitration is usually based on the parties' legal rights and obligations, not their business interests.

Contrary to popular belief, an arbitrator does not (or at least should not) just "cut the baby in half". They find the facts based on the evidence. They apply the relevant law to those facts. They then determine the parties' legal rights and obligations and resolve the dispute as the law requires, based on those facts.

That process presents the opportunity for a party to win the dispute - to "hit a home run." Of course, that necessarily also
presents the risk of losing - of being the pitcher who gives up that home run.

Arbitration has significant potential advantages.

The parties have (at least) input into the choice of their decision maker. That can give comfort that the result will be at least acceptable, if not necessarily ideal.

Arbitration has tremendous procedural flexibility. Experienced counsel can tailor its procedures to focus on exactly what is needed to resolve the particular dispute. That can lead to significant cost and time efficiency compared with litigation.

However, the fundamental nature of arbitration involves one big potential disadvantage: the possibility that the decision imposed on the parties by the arbitrator is unacceptable to one (or both!) of them. There's no avoiding that.

It's inherent in the nature of arbitration (and litigation), as opposed to negotiation or mediation.

In addition, while arbitration procedures are very flexible, and promote efficiency, they are much closer to those of litigation than those of negotiation or mediation. Cost and time efficiency therefore suffer by comparison.

Litigation

Litigation is fundamentally the same kind of process as arbitration: a neutral has the power to impose a legally binding resolution on the parties. But instead of the parties agreeing on that neutral, they're appointed by the state, in the person of a judge.

It is crucial to understand that litigation, warts and all, is our society's default dispute resolution mechanism. If the parties don't agree on another mechanism, their dispute will be resolved by litigation, in any jurisdiction whose courts are willing to take it on.

While litigation has its place, it should be obvious from a consideration of its advantages and disadvantages that it is not usually the best option.

Litigation generally has only one significant advantage: certainty. As with arbitration, the process will almost certainly resolve the parties' dispute. But that's it.

There are generally no other advantages to litigation.

On the other hand, there are a host of disadvantages. The parties have essentially no input into their choice of decision maker.

Their dispute is resolved by whoever the relevant court's bureaucracy assigns to it. In Canada, where (outside Toronto) there is no specialized roster of experienced commercial judges, that can be a real problem in complex commercial cases.

As with arbitration, there is the possibility of an unacceptable decision being imposed.

Litigation procedures, despite some recent tinkering designed to make them more efficient and user-friendly, are relatively inflexible. The mandatory disclosure of relevant documents to the other parties, which is such an essential part of litigation, can be a real burden in the email age.

Litigation is usually the most expensive and time consuming dispute resolution mechanism.

Compared to arbitration, there is a lack of finality. Either party can appeal a trial decision as of right. Then the parties have to do it all over again (not quite) in an appellate court. Enforcement of a judgment around the world is more difficult than enforcement of an arbitral award.

Absent a sealing order, which the courts are reluctant to grant in commercial cases,the parties' dispute will be played out in public, with all the evidence, arguments and results available to anyone who cares to look (and circulate on the internet), including the media.

All in all, not a pretty picture. One to be avoided if possible.

Conclusion

Negotiation and mediation on the one hand, and arbitration and litigation on the other,are fundamentally different kinds of dispute
resolution mechanisms. Each has distinct advantages and disadvantages.

Business people and corporate house counsel should carefully consider the kinds of dispute which are likely to arise under the contracts they negotiate, and chose a dispute resolution mechanism (or combination of mechanisms) which is best suited to resolve those kinds of dispute.

If they don't chose wisely, they may end up being committed to a mechanism which is inappropriate for the disputes which do arise.

If they don't chose at all, then by default they chose litigation. There is usually a better choice.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

There are two issues regarding Franchisor control that have bubbled under the surface for a number of years and are now beginning to become significant to Franchisees and Independent Franchisee Associations alike.

Since a Federal judge ruled that Coverall North America must pay triple damages to hundreds of workers misclassified as franchisees instead of employees, everyone in franchising is now aware of the dangers of a franchisor who has too much contractual control.

The control issues are:

1. Operational Control

2. Supplier Control

Not coincidentally, these issues have become more prevalent and evident with the advent of strong Independent Franchisee Associations.

As the Franchisors continue the erosion of certain aspects of a Franchisees Business as well as the ability of an Association to exist, they may experience some dire consequences if not careful.

Note the simple graphic below:

<----------------------------------{ **** }------------------------------------------------------------------------------------------------------------------------------------->

^ ^ ^

Independent Operator Independent Contractor Employee

The scale above represents at the furthest left hand point, the ability of the Franchisee to do what ever he/she wants with very little direction from the franchisor. At the furthest point on the right hand of the scale represents the Franchisee as strictly employee and no other distinction.

The 'window' (represented by the brackets on the line) shows where the Franchisor has been on the scale.

Operational Control

The Franchisor has the exclusive rights and fiduciary duty to protect its Trademark and Brand. This certainly includes standards of operations, protecting trademarked signage, logos and products.

Franchisees sign an agreement with the Franchisor with the expectation that the Franchisor is an 'expert' ith the chosen field. "The Franchisor's Plan" will change according to the market place.

Franchisees should also enter their agreements knowing that reinvesting in the business reasonably will be a part of keeping up with the competition.

The graphic above is now showing a much different picture. The "window" is gradually and some cases dramaticlly toward the right side of the bar and is being driven by actions on the part of the Franchisor. In the case of some franchised systems, becoming a franchisee is no more that purchasing a job.

As these sifts to the right of the 'line' continue to occur, it is incumbant upon Franchisees and their Associations to become extremely aware of the State and Federal guidelines that determine whether the Franchisee is truly an Independent Operator, and Independent Contractor or an Employee.

It is very tempting in the current employment environment which features legislation such as the Fair Pay Act, Insurance Reform, Employee Free Choice Act (not yet passed and somewhat on the back burner) and others for not only Franchisors, but Small Business in general to attempt to avoid compliance by classifying their workers as Independents.

They are not only relieved of the legal issues, but don't pay FICA, Workers Comp, Unemployment Insurance and the like. There is only on small problem; It's Illegal!

These issues that have been brought to the forfront regarding the Coverall case as well as the Federal Express Drivers complaint.

Supplier Control

The issue of supplier control rests squarely on one question; "Does excercising strict controls over certain products and services protect the Brand and the Brand Image?"

The Franchisor's supply chain management in most systems have standards, supplier facility inspections, quality control metrics (checked frequently from the manufacturer) and the ability to protect itself from fragmentation, inconsistency and dereliction due to inferior or harmful products.

The Franchisor's supply chain management team must be allowed to exercise control over products which would destroy a brand if due diligence were not done.

It is in the best interest of all Franchisees to forbid the use of products that would harm customers or in some cases cause death.

However, what seems to be occurring is a not so subtle choice on the part of Franchisors to 'grab' control of products and services that have nothing whatever to do with Brand protection and the avoidance of harming the consumer.

Newsletter, work shoes, floor mats, individual websites, certain forms of business insurance and other have all been grabbed by the franchisor.

For individual Franchisees and particularly for the Independent Associations, the willingness of the Franchisor to take control of these items has encroached on the remaining ability of the operator to influence their own unit economics and have taken away from the Associations a very important source for funding.

You will see more information in the very near future as well as collaborative solutiuons in the future.

More and more issues surrounding Franchisor control will continue to be brought to light and we intend to keep you informed and even look at actions that can be taken in the future.

(This is a reprint and update of an article the late Steve Ellerhorst wrote 3 years ago, and it still rings true today.)

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.

Franchises are mostly created or started by a single founder.

The founder faces an interesting strategic challenge in persuading enough franchisees to voluntarily pay for & add value to the franchisor's property - the franchisor's trademark and confidential information.

1) If enough do, then we will all be better off because we have formed a brand.

2) However, some will not live up to the brand's standards and free-ride on the brand's reputation.

If enough of us expect that others will free-ride, we won't live up to the brand's standards either.

The brand may just flounder along.

(This type of problem is known by many names: social dilemma by social psychologist, the collective action problem by game theorists, the social contract by philosophers, the buy-in problem by management theorists, and the tragedy of the commons by political theorists. )

What sort of personality gets the job done?

How does the franchise system get buy-in from the franchisees? What happens when the founder leaves the system?

How does the franchisor continue to get buy-in from the franchisees?

Some social psychology research on social dilemmas suggests that the franchisor must be dominant and aggressive to lead.

A social dilemma exists when we know that if some us coordinated, we would be better off. But, we also know that most us think that not enough of us will coordinate and so any benefit to coordination is at best illusory and perhaps a trap. So, some argue, we need to be forced, coerced or bullied into joining.

Groups need to be lead by a dominant and assertive leader in order for the benefit of coordination to be real & exist, is the claim.

Robert Livingston and many others argue for this conclusion, lending support to benevolent dictator picture of a franchisor founder.

"Being kind and self-sacrificing will get you plenty of friends, but won't help you win a corner office", argues management professor Robert Livingston.

The altruistic are typically seen as good people, but not dominant and aggressive enough to lead, Livingston's research shows.

"On the one hand, generous individuals are admired," he says.

"On the other hand, they may be perceived as feeble 'bleeding hearts' who lack the guts to make tough decisions.

And, we need dominant and aggressive leaders because:

In social dilemmas "when groups had to compete against each other, dominant individuals rose to the top while benevolent people were least likely to be elected."

(It is well worth listening to Livingston explain his theory of leadership in more detail.)

On the other hand, Tom Schelling explained, almost 35 years ago, the solution to a social dilemma didn't need a certain personality type. The solution required the existence of a group disciplined enough that, even though resentful of free-riders, its discipline could be profitable for the group (though even more profitable for those who stayed out.)

A combination of discipline but resentment. Not altruism, not dominance, but a combination of discipline with a type of resentment focused on the free riders would stop the group from unraveling.

How can we decide between Schelling and Livingston? One idea is to use a negotiation training exercise & a variety of social dilemmas to record people's emotional & immediate responses.

Trainers in leadership have been using social dilemma exercises to produce various "Aha" moments, for quite some time.

One such exercise is called "Win as Much as You Can".

What is Next?

I want to step through an analytic discussion of the Win as Much as You Can exercise. (Only by playing it with real people can you get the experiential content. In most cases, the group fails to coordinate, and remains in the state of nature - and more so with dominant and aggressive leaders!)

Then, I am going to suggest an alternative exercise and provide reasons why, if Schelling is right, this new exercise should provide an "Aha" moment about how discipline and resentment can hold a group together.

In what follows, there will be -for some too much- use of calculation, simple graphs and decision theory. So let me give away the conclusion quickly -right after the description of the game.

Win as Much as You Can Game.

You and three others each have two choices, play Red or Blue, R or B. What each of you gets depends on what the others play and your choice, according to this table.

Group Individuals

4 Reds - Red player gets -1.

3 R, 1 B - Red players gets 1, Blue Player -3.

2 R, 2B - Red players get 2, Blue Players -2.

1R, 3B - Red player gets 3, Blue Players -1.

4 Blues - Blue Players get 1.

If this game is played, say for ten rounds, each player by playing only B, could win 10. Yet, in the thousands of exercises trainers have completed, almost no one or group gets to this outcome. (In most exercises, there are two bonus rounds, which simply complicates the scoring for no good theoretical reason.)

The group coordination of 4B's almost never happens. Even if it was expected, then some expect others to cheat and play R, and so they would play R first to "protect" themselves against the cheaters they have become.

Self fulfilling prophecies are at the heart of many a bad decision in which we start to expect the other person to act in a way contrary to our wishes - "seeing" them this way makes it more likely that they see us as acting contrary to some of their wishes. And so it goes.

But not always, and that is a bit curious. Schelling's insight was: not all of us, at the same time, have to see everyone else as a possible threat which unravels the group's coordination.

Is Schelling right?

Here is what I will show. If he is, then by changing the (1R, 3B) payoff to Red player gets 3, Blue Players get 0, two things should happen.

First, more groups should have higher scores in the modified Win as Much as You Can Game.

Second, the subgroup of 3 Blue players that coordinates should experience both discipline and resentment, but not enough resentment to unravel the group.

By coordinating, the 3B players can lift themselves out of the state of nature, in which they all receive -1, to 0, for a gain of 1, but the R free-rider gains 3. Note, Schelling is not saying that this subgroup has to form, only that if a subgroup does, it will be this one.

And if it stays together, discipline and resentment will run together. Discipline need to stick together and resentment against the free-rider, who plays R.

(If you are a trainer, you can run both exercises, report back - all without having to do any of the calculations, which follow below.)

Justification for the Change in Payoffs

For the intellectually curious, we push on.

It seems unlikely that the group will play 4 B's together. Further, if one person should correctly forecast that the group was leaning to 4 B's, it would be in their interest to act as if they were going to play B, just in order to play R at the last possible moment. Much like a good poker player bluffing the table.

If only one succeeds at this strategy - this success will invite mimics. Soon the coordinated strategy of 4 Bs will completely unravel.

The remainder of this article will provide reasons justifying why the small change in the original game should bring about some subgroup coordination.

1. Transforming the Win as Much as You Can Exercise into a several Multi-Person Prisoner's Dilemma Game.

First, we will take some time to show that the Win as Much as You Can game is a combination of several special social dilemmas, Schelling called these "Multi-Person Prisoner's Dilemmas", or MPDs.

A prisoner's dilemma is a social dilemma in which the unraveling is caused by enough people reasoning using a principle from decision theory: If no matter what everyone else does, I would be better off choosing R over B, I should always chose R.

There is much to be said for this rule. Some have elevated it to a canon of rationality. If others disagree with the rule, then when it is available, their decisions make those who follow the rule better off.

The well-known problem with the rule is that its use in a multi person prisoner's dilemma produces dismal results.

(A word is needed about the diagrams that follow. I usually make mistakes drawing them - especially when I "know" how they should turn out. To avoid making mistakes, I have created a checklist or algorithm, which helps me. For those more talented than I, this checklist will be too tedious. However, for you and I, I believe it to be helpful.)

The Payoff Algorithm

Step 1. Identify the choices and individual can make, R or B.

Step 2. Identify the outcome the group can arrive at, counting each outcome as distinct based upon whether the individual played R or B.

Only Step 2 needs an illustration. Consider the pattern 3R, 1B. This is two outcomes - if you played Red, the outcome is 1, while if you played Blue, the outcome is -3.

Step 3. Count the outcomes in a systemic manner and plot.

There are eight outcomes in this case and not 10 because 2 patterns 4R and 4B have only one outcome, while all the other patterns have two outcomes.

How should we label these eight outcomes?

One way is to pick a variable k, running from 0 to 3, where k is the number of other individuals playing B. The outcome O_2k+1 is the outcome where you play B and k other individuals also play B. The outcome O_2k+2 is the outcome where you play R and k other individuals play B.

The outcomes range from O₁ to O₈.

O₁ is the outcome where k=0, nobody else plays B, but you do. The payoff to the B player if the pattern is (3B, 1R) is -3.

O₂ is the outcome where k=0, nobody else play B, and neither do you. The pay off to the R player if the pattern is 4R is -1.

For, k=1, 2k+1 =3 and 2k+2=4.

O₃ is the outcome in which one person plays B, and so do you. The payoff to the B player if the pattern is (2B, 2R) is -2.

O₄ is the outcome in which one person plays B, and you don't. The payoff to the R player if the pattern is (1B,3R) is 1.

Let's summarize all of the outcomes.

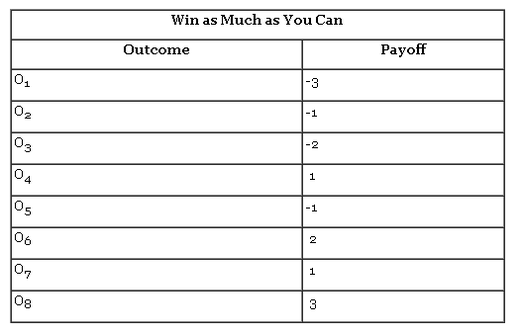
We can now draw a diagram, with k on the X axis, and the Y axis reflecting the payoffs of B and R, given k people have chosen already to play B.

There are two easy things to read off the graph. (This is not a single MPD because the lines are not straight.)

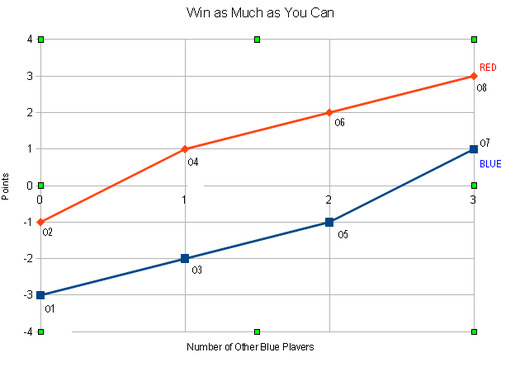
1. Red dominates Blue - for no k, or group of individuals, would be better to be part of the k group of B players. For each k, you are better off being a R.

2. But O₂, 4R, is a bad place for everyone compared to O₇, 4B.

(For those wondering if this hasn't been a lot of work to recover some obvious points, there had better be a reward for making it this far!)

The reward is to focus on O_5, and the horizontal line we can draw from O₂ - the state of nature. The horizontal line is the payoff in the state of nature, any outcome above that -short of complete group coordination- is a group whose discipline is both profitable to join, yet profitable to leave.

The outcome O₅ or the pattern (3B, 1R) has a payoff of -1, but is tantalizing close to the optimum 4B, O₇.

If 3 players played B, how much harder would it be to get the last person on board?

In this case, it should be very hard. For the R player, the pattern in O₅ is the outcome O₈ - how can you persuade him or her to accept 1 instead of 3?

Thus, if three players can coordinate their actions by playing B, their payoff isn't greater than the state of nature, O₂. Partial coordination doesn't pay and it doesn't make it more likely that full coordination is reached. Two players cannot do any better.

But, what does the diagram look like if we change O₅? Let's just move it above the horizontal line and give the B players 0 instead of -1, at O_5'.

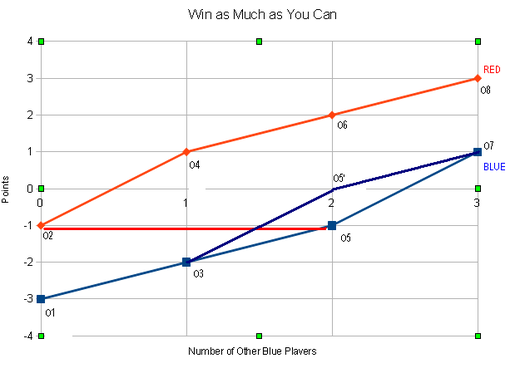
Now, the coalition of 3B in this new game satisfies Schelling's criterion:

"The smallest disciplined group that though resentful of free-riders can be profitable for those who join (though more profitable for those who stay out)."

Is it true? Can this simple change in pay-offs actually make a difference or is this a theoretical possibility only? What do you think will happen if this training exercise is played with both games instead of just the standard game?

We need to try it and see if Schelling's analysis bears fruit.

Did you like this? Why not click here and subscribe to Webster's Strategic Stories.

Your friends and competitors are already reading and learning more business strategy. Why not you?

Kids still read books, but nobody uses Quill pens, the Tennessee Court of Appeals in reversing the lower court and finding that nexus exists even without agents or a physical presence.

Remember Scholastic Book Club? Those were the books you ordered in grade school and your teacher placed the order for the class. A few weeks later, a package arrived and teacher gave you the books.

Sort of like Amazon but a lot easier since you could guilt-trip your parents into buying you some cool mystery paperbacks.

Book clubs may be passe, but empty state coffers are current news.

The State of Tennessee wanted to charge Scholastic sales & use tax.

The company argued that it had no presence in Tennessee, and that teachers who took the book orders were agents of the students. The company also argued that it did not place demands on state services.

In particular, the company relied on Quill v North Dakota (1992) and said that there was no tax nexus that would be recognized under US Supreme Court jurisprudence on the matter.

On appeal, the nexus issue was presented:

Whether the activities of in-state schools and school employees on behalf of an out-of-state seller that enable the seller to establish and maintain a market in Tennessee create sufficient nexus with Tennessee under the Commerce Clause of the United States Constitution to support an assessment of Tennessee sales and use taxes against the seller.

The court opinion is troubling for franchisors. The court held that Quill was dispositive and nevertheless managed to find tax nexus:

the issue in this case is not whether Tennessee teachers may be considered agents of SBC, but whether SBC's connections with Tennessee's schools and teachers establishes a "substantial nexus" such that the Commissioner's assessment may be sustained under the Commerce Clause

The court found:

In short, SBC has created a de facto marketing and distribution mechanism within Tennessee's schools and utilizing Tennessee teachers to sell books to school children and their parents. Contrary to SBC's assertion that it uses no public services in Tennessee, this State's school facilities and teachers are, in large part, funded by taxpayer dollars. We agree with the Commissioner that SBC's connections with its customers in Tennessee do not fall with the narrow safe harbor provisions affirmed in Quill Corp

Of particular interest to franchisors is the court's statement that Quill stands for the proposition that contact may only be via "common carrier or the United States mail."

By the reasoning of the Tennessee appellate court, franchisors with sales agents and support/compliance audits within the state are outside the "narrow safe harbor" of Quill.

Unlike the schoolteachers in Scholastic, the local representatives of franchisors are not working without compensation; in fact their compensation is often tied to a percentage of what the franchisor is earning. It is true that the appellate court noted the taxpayer support of schoolteachers, but a reading of the Opinion suggests that this was not the basis for the decision and more in the nature of dicta than a necessary element of finding nexus.

The court ruling is a roadmap for SCOTUS to gut Quill without actually overturning precedent, and the Scholastic reasoning is likely to be closely read when and if Quill is challenged at the high court.

For the 5 Most Fascinating Stories in Franchising, a weekly report, click here & sign up.